Derivative graph of y=x^2 256426-Derivative graph of y=x^2

The derivative of a function y = f(x) of a variable x is a measure of the rate at which the value y of the function changes with respect to the change of the variable x It is called the derivative of f with respect to x If x and y are real numbers, and if the graph of f is plotted against x, the derivative is the slope of this graph at each point Problem Find the derivative of y =x^29 Plot the function and the derivative Solution Lets say you had the graph of just x^29 (without the absolute value, represented by the orange line in the graph below) that would look like the graph of x^2 shifted down 9 units y=(x2)(x2)(x4) graph{(x2)(x2)(x4) 10, 10, , } Fist we find the roots of the equation, ie the values of x st y=0 y=0 => (x2)(x2)(x4)=0 (1dp) These values of x correspond to the critical points (or turning points) we now use the second derivative to determine the nature (max, min or point of inflection) of these

Derivatives

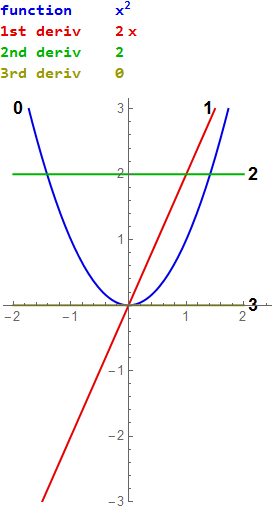
Derivative graph of y=x^2

Derivative graph of y=x^2- Explain how the sign of the first derivative affects the shape of a function's graph State the first derivative test for critical points Use concavity and inflection points to explain how the sign of the second derivative affects the shape of a function's graph Explain the concavity test for a function over an open interval If you do a simple test visually say, the first segment of your reference graph is concave up and positive slope The positive slope notifies that the graph of the derivative will be in the positive terminal The concave up quality of the initial part of the graph assumes that the derivative is increasing

Ac The Second Derivative

Answer to Find the derivative, dy/dx if y=x^2 By signing up, you'll get thousands of stepbystep solutions to your homework questions You canPart 2 Graph Then find and graph it Graph of Graph ofCA The graph has a local minimum at x = 4

 Here are the derivatives of the 3 functions given above 1 Quadratic (parabola), `y=x^210x1` Derivative `dy/dx=2x10` 2 Cubic, `y=0015x^3025x^49x047` Derivative `dy/dx=0045x^5x049` 3 Quartic `y=x^415x^36x^235x3` Derivative `dy/dx= 4x^345x^212x35`For example, for y = x 2 the derivative wrt x is y = 2x How can I do this ?A Quick Refresher on Derivatives A derivative basically finds the slope of a function In the previous example we took this h = 3 14t − 5t 2 and came up with this derivative ddt h = 0 14 − 5(2t) = 14 − 10t Which tells us the slope of the function at any time t We used these Derivative Rules The slope of a constant value (like 3) is 0;

Suppose the derivative of the function y=f(x) is y' =(x 2)²(x – 4) At what points, if any, does the graph off have a local minimum, local maximum, or point of inflection?19 Questions Show answers It's the average rate of change of y=3x^24x2 on the interval 2, 1 When you plug a given point into the derivative of a function, you get this Use the definition of the derivative (aka the limit process) to find the derivative of y=x^29 (be ready to show work)Ex Find the directions in which the directional derivative of f(x, y) = x2 sin(xy) at the point (1, 0) has the value 1 ( answer ) Ex Show that the curve r(t) = ln(t), tln(t), t is tangent to the surface xz2 − yz cos(xy) = 1 at the point (0, 0, 1) Ex A bug is crawling on the surface of a

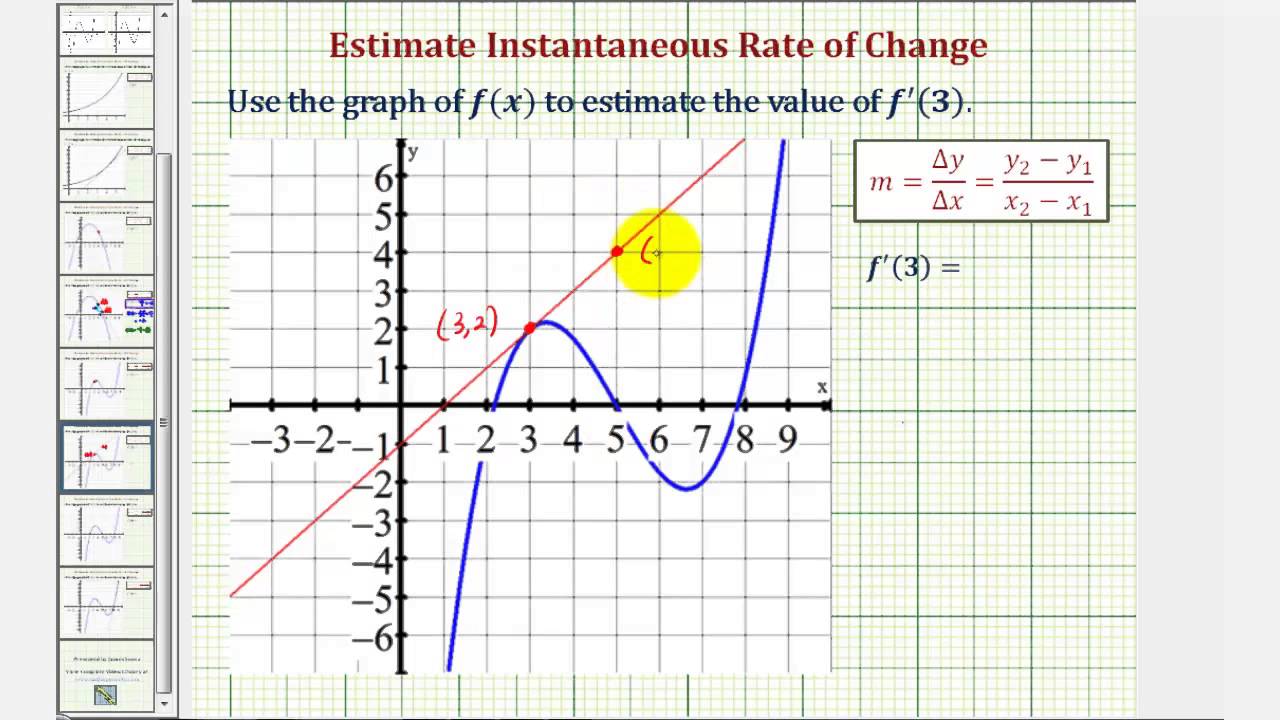
Ex 3 Estimate The Value Of A Derivative At A Point On A Graph Using A Tangent Line Youtube

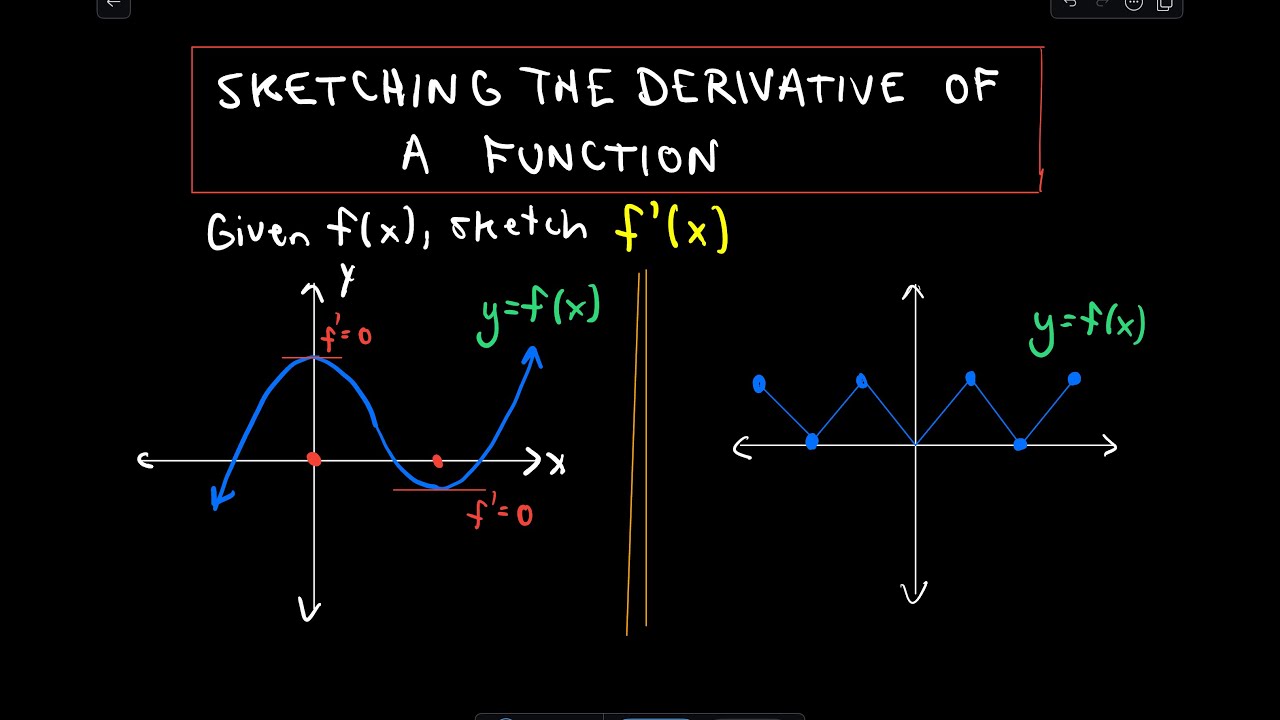
Sketching The Derivative Of A Function Youtube

So here's a picture of function 2, the derivative, or the slope, which I was calling s So that's the s function, against x x is still the thing that's varying, or it could be t, or it could be whatever letter we've got And the answer was 2x for this function So if I graph it, it starts at 0, and it climbs steadily with slope 2 So that's a(a) Graph the function $ f(x) = x^4 3x^3 6x^2 7x 30 $ in the viewing rectangle 3,5 by 10,50 (b) Using the graph in part (a) to estimate slopes, make a rough sketch, by hand, of the graph of $ f' $ (c) Calculate $ f'(x) $ and use this expression, with graphing device, to graph $ f' $ Compare with your sketch in part (b)1 Graphing the Derivative of a Function Warmup Part 1 What comes to mind when you think of the word 'derivative'?

Http Www Homepages Ucl Ac Uk Zcahc79 Math6103 Lecture Notes 6103 Lecture10 Pdf

Www Apsva Us Wp Content Uploads Legacy Assets Washingtonlee 3163bc16 Derivative Graph Notes Pdf

 The graph of $f$ can also be described as the set of points in the $x$$y$ plane that satisfy $y=x^2$ Then, we can write $\frac{dy}{dx}=2x$ (not $\frac{dx}{dy}=2x$ ), which has the same meaning as $f'(x)=2x$ , but tries to capture a slightly different ideaDerivative Calculator Step 1 Enter the function you want to find the derivative of in the editor The Derivative Calculator supports solving first, second, fourth derivatives, as well as implicit differentiation and finding the zeros/roots You can also get a better visual and understanding of the function by using our graphing toolThe Derivative Calculator supports computing first, second, , fifth derivatives as well as differentiating functions with many variables (partial derivatives), implicit differentiation and calculating roots/zeros You can also check your answers!

Solve Tangent Lines Problems In Calculus

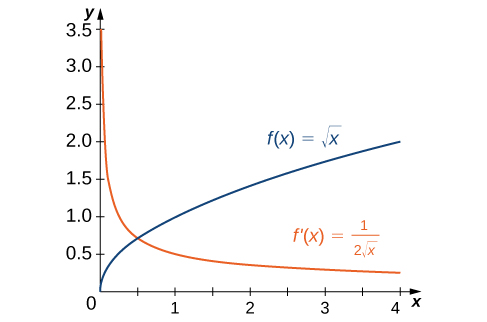
3 2 The Derivative As A Function Calculus Volume 1

The slope of a line like 2x is 2, so 14tMath 1300 Calculus I The Derivative Function 1The purpose of this problem is to see how to construct a derivative function one point at a time by looking at a graph Background review estimating derivatives, one point at a time The derivative of a function at a point represents the slope (or rate of change) of a function at that pointPartial Derivatives 1 Partial Derivatives Just as df dx is the rate of change of f(x) when x is changed, so the derivatives of f(x;y) are the rates of change of the function value when one of the variables is changed Since there are two variables to choose from, there are two derivatives,

Content Transformations Of The Parabola

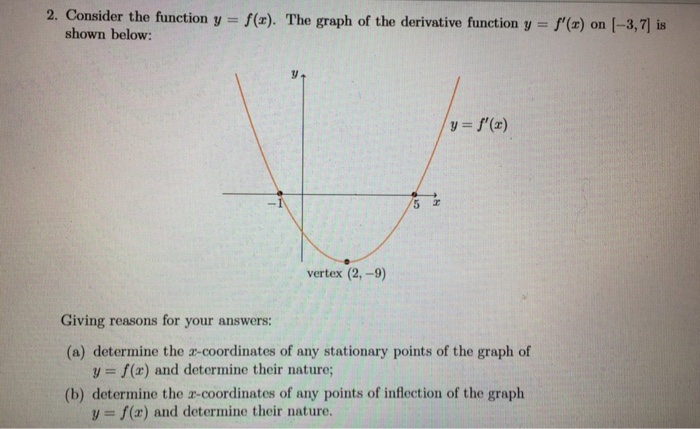
2 Consider The Function Y F X The Graph Of The Chegg Com

Implicit differentiation, derivative of x^y=y^xcheck out calc 1 life hack, https//youtube/ZI8jF5AVWccheck out how to find the parametric equations httpsDivide 0 0 by 4 4 Multiply − 1 1 by 0 0 Add 0 0 and 0 0 Substitute the values of a a, d d, and e e into the vertex form a ( x d) 2 e a ( x d) 2 e Set y y equal to the new right side Use the vertex form, y = a ( x − h) 2 k y = a ( x h) 2 k, to determine the values of a a, h h, and k kDerivative Calculator Enter a function to differentiate Variable Order 1 2 3 4 5 6 7 8 9 Derivative of (xy)/ (xy) by y = (2*x)/ (y^22*x*yx^2) Show a step by step solution Draw graph Edit expression Direct link to this page Value at y= Derivative Calculator computes derivatives of a function with respect to given variable using analytical differentiation and

Calculating The Derivative Of Ln X 2 Video Lesson Transcript Study Com

3 2 The Derivative As A Function Mathematics Libretexts

1234567891011Next

Incoming Term: derivative graph of y=x^2,